Abstract
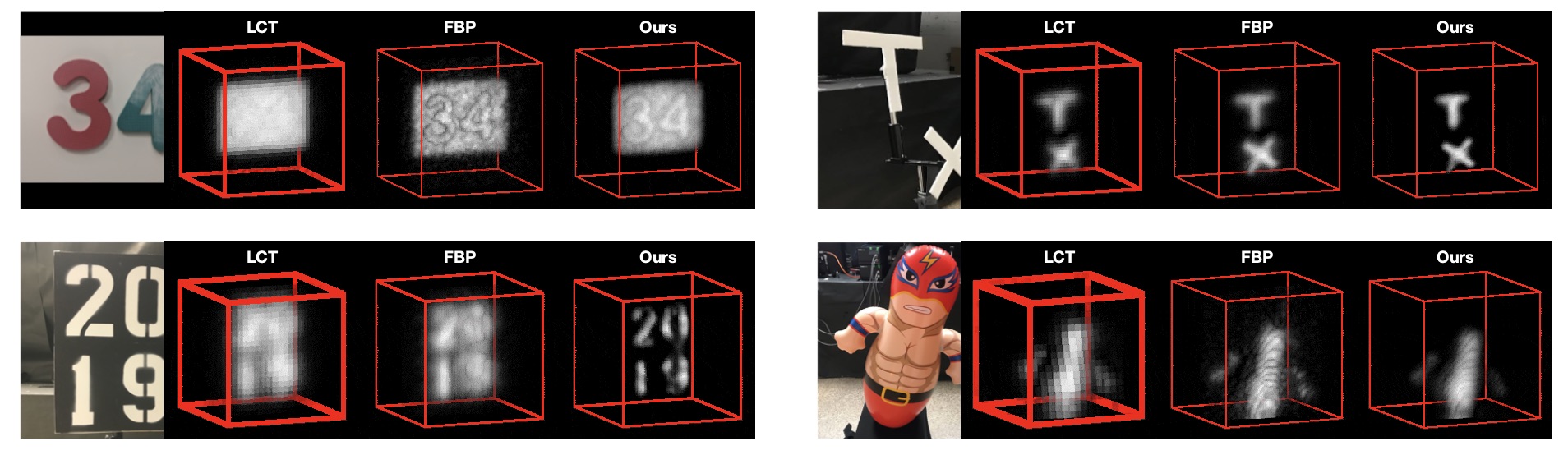
Non-line-of-sight (NLOS) imaging aims to reconstruct scenes outside the field of view of an imaging system. A common approach is to measure the so-called light transients, which facilitates reconstructions through ellipsoidal tomography that involves solving a linear least-squares. Unfortunately, the corresponding linear operator is very high-dimensional and lacks structures that facilitate fast solvers, and so, the ensuing optimization is a computationally daunting task. We introduce a computationally tractable framework for solving the ellipsoidal tomography problem. Our main observation is that the Gram of the ellipsoidal tomography operator is convolutional, either exactly under certain idealized imaging conditions, or approximately in practice. This, in turn, allows us to obtain the ellipsoidal tomography solution by using efficient deconvolution procedures to solve a linear least-squares problem involving the Gram operator. The computational tractability of our approach also facilitates the use of various regularizers during the deconvolution procedure. We demonstrate the advantages of our framework in a variety of simulated and real experiments.

Key Idea
Our main technical result is to show that, under certain assumptions on the imaging geometry, the Gram of the NLOS measurement operator is a convolution operator. This result advances NLOS imaging in three important ways. First, it allows us to efficiently obtain the ellipsoidal tomography reconstruction by solving an equivalent linear least-squares involving the Gram operator: As the Gram operator is convolutional, this problem can be solved using computationally-efficient deconvolution algorithms. Second, it provides a theoretical justification for the filtered backprojection algorithm: We can show that filtered backprojection corresponds to using an approximate deconvolution filter to solve the problem involving the Gram operator. Third, it facilitates the use of a wide range of priors to regularize the NLOS reconstruction problem: The convolutional property of the Gram operator implies that the corresponding regularized least squares remain computationally tractable.