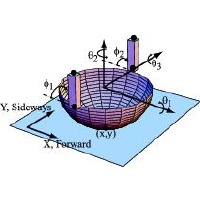
A flipped turtle is stuck because it’s feet do not touch the ground. One way of escape is to rock itself on it’s shell so that it rights itself. Alternately, can the turtle locomote in it’s flipped configuration merely by swinging the legs and rocking back and forth? Similarly, what happens when a robot is high-centered? How can it move in such a situation? My doctoral work has “discovered” this novel locomotion technique for high-centered robots, a locomotion we call emph{legless locomotion}. The high-level goal of this project is to investigate escape/recovery strategies when robots get stuck (mobile-robot errors).

To simplify the high-centered-robot problem, we built a prototype called Rocking and Rolling Robot (RRRobot). It is “high-centered for life”, that is, its legs don’t touch the ground in its nominal position. We show in a recent paper that it is possible to locomote RRRobot in the plane just by wiggling its short legs, i.e., the robot locomotes by transferring energy and momentum to produce a rocking and rolling body motion. This rocking motion when coupled with the nonholonomic contact constraints produces incremental translation. An important aspect of this locomotion is that RRRobot’s inertia varies with leg configuration.